Why does insulation work at all?
kurtgranroth
| Posted in General Questions on
I’m trying to learn more on how insulation works, but articles on the subject tend to be either far too basic or far too technical — I’m looking for a mid-level understanding.
To start, heat flows from an area of higher heat to an area of lower heat and the rate of flow is related to the magnitude of the difference in the two temperatures (the bigger the delta, the faster heat flows). In general, insulation works by slowing down that heat flow but does not stop it. Increasing the R-value (or lowering the U-value) of the insulation will slow down the heat transfer even more.
The specific way that insulation slows the heat transfer down depends a lot on the type of insulation in play. All reduce or eliminate convection and use materials and layers with low thermal conductivity and emissivity. In doing this, they will all also necessarily temporarily “absorb” the thermal energy as it makes its way slowly through the material.
Let’s say we have a constant heat source radiating against an insulation layer. On the other side is an area with a much lower temperature. If there is a sufficient insulation layer compared to the magnitude of the heat source, then the lower temperature area will not have an appreciable heat rise (at least at first) as the insulation absorbs the heat and only very slowly allows it to flow out.
But… let’s allow that to go for longer. The insulation slowed the heat transfer down but since it doesn’t/can’t stop it, that heat will eventually make it out into the low heat area. In fact, if the heat source is constant, then the heat will always flow towards the low heat side. EVENTUALLY, wouldn’t 100% of the heat that enters the insulation flow into the low heat area?
Given that, I guess I don’t know how insulation actually does any useful work, other than giving you a little time delay before the heat starts making it through since all of it eventually will make it through. It almost sounds like you could just forgo insulation entirely in cooling climates since all of that incoming heat will make it inside regardless of how much insulation you have!
Except… insulation absolutely does work and it works very well even in cooling climates. That suggests that I’m missing something very fundamental! What is it that I’m missing?
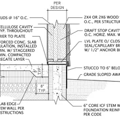
GBA Detail Library
A collection of one thousand construction details organized by climate and house part
Search and download construction details







Replies
> eventually
It's usually about the rate of heat transfer, not stopping transfer. So time matters and "eventually" isn't relevant.
Okay, but why isn't it relevant?
An analogy. Say you are standing under a faucet that is pouring water on you. You then take a bucket with a small hole in it and put it between you and the faucet -- this reduces the water flow. In the first case, you'd get soaked very quickly and the in the latter case, it will take longer to get soaked... but you will be equivalently soaked in either case, eventually.
What you need to realize is that times has a value. You want your house to stay around 21C all the time. If you are loosing less heat, it takes less heat to replace that loss and to keep the house at 21C. The goal is to keep a constant temperature not to provide X amount of heat to your house so slowing the heat loss is very important.
If you were taking a bath and the water was leaking, the bigger the hole, the more water you'll need to keep the level constant. The same way a house would require more heat to keep its temperature constant.
Heat is not the same as temperature. The more heat your house loose, the faster its internal temperature will drop and the more heat you'll have to produce to prevent it from dropping.
To put it another way, you house will leak heat, and given the properties of heat, it's virtually impossible to create a perfect barrier to heat transfer (unlike trying to contain liquids). But you can slow it down by multiple orders of magnitude.
Insulation slows the movement of heat, reducing the amount of energy per hour or day required to make up for the loss.
That's why heat and cooling loads as well as equipment capacities are typically expressed per hour.
>”Okay, but why isn't it relevant?”
Because energy transfer is a RATE, with a TIME component. If your insulation slows heat transfer 50%, that reduces your heat loss from (making up some numbers for an example here) 50,000 BTU/hour to 25,000 BTU/hr. That means you’re using half as much energy to replace the lost heat per unit time.
Since you’re constantly putting in heat to replace what is lost, slowing the rate at which the heat is lost also reduces the total amount of heat being lost in each unit of Time. You need to think in terms of discrete units of time. You have two situations here:
1- steady state, with heat input maintaining a constant temperature inside the space. If you have a typical on/off cycling heat source, the cycles will be shorter and/or less frequent to replace the lost heat to maintain a constant interior temperature. With a modulating heat source, the total heat output will be reduced to match the rate of loss through the insulated wall. In both cases, the amount of input energy require to maintain the constraint interior temperature is reduced.
2- no input energy. In this case, the interior temperature is allowed to fall until equilibrium is reached with the exterior temperature. Energy always moves from areas of higher concentration to areas of lower concentration, so the interior space will gradually cool off. The insulation in this case serves the same function of slowing the rate of heat loss, but now it slows the time it takes to reach equilibrium with the exterior temperature. In any given unit of time, a lower amount of energy is lost, which means the interior temperature drops more slowly.
You have been thinking in terms of infinite time. In that situation, you use either infinite energy, or your interior temperature drops to match the exterior temperature. In reality, infinite timescales don’t matter — we care about FINITE time scales. In a finite time scale, the slowing of heat loss due to the insulation results in a reduction of energy required to maintain a constant temperature over a finite period of time. It comes down to the PER HOUR part of the BTU/hr unit.
Bill
Okay, so I was definitely missing that timescale matters quite a bit. The missing pieces are coming together... but I'm not yet 100% of the way there.
I'm here in AZ and so I think of heat transfer exactly opposite to how most people do -- it's not something to "keep in" but is instead something to "keep out". My attic is around 160 degrees during the day and around 100 degrees when the sun goes down, roughly half of the year. I keep the A/C at 80 degrees so the heat flow will be always coming in for at least 6 months straight. The walls are similar but there is typically at least an hour of the day where the temperature is at stasis so the pressure is only for 23 hours a day during those 6 months (and notably less pressure than the ceiling at all times).
So if I have a lot of insulation in the attic, then I will see a lower RATE of heat coming into the house and (thankfully), my A/C can spend significantly less energy pumping that heat back out. The more I can decrease the thermal transfer rate, the less energy I use cooling my house. Nice.
But while this heat pressure isn't infinite, it is constant for many months at a time. If all of that energy is being temporarily absorbed into the insulation and it's being transferred at a low rate, then that would suggest that the insulation would contain a huge amount of thermal energy by the end of the heating season and all of the heat will still need to go somewhere.
Two thoughts there. The first is that maybe the insulation does have that much "stored" thermal energy, but as we swing into winter, then the flow can reverse some of the time, and maybe it can finally transfer the spring/summer/fall energy out, but to the outside rather than in?
The second is that maybe not as much thermal energy as I thought is being "stored" in the insulation in the first place? That is, I picture the heat entering the insulation and staying there until it slowly ekes out. That would likely require a material with very high "thermal mass" (heat capacity?) and most conventional insulation materials don't have that. So in that case, even though the heat pressure on the insulation is relatively constant for a long time, the rate that it ENTERS the insulation is also slow and so the amount of heat in the insulation at any given time that can make it through is relatively low?
Insulation doesn't store heat, it just slow conductive heat losses. You are confusing thermal mass and thermal insulation. If you want to store heat, what you want is thermal mass like a concrete wall.
>"But while this heat pressure isn't infinite, it is constant for many months at a time. If all of that energy is being temporarily absorbed into the insulation and it's being transferred at a low rate, then that would suggest that the insulation would contain a huge amount of thermal energy by the end of the heating season and all of the heat will still need to go somewhere."
The "heat pressure" isn't even constant over a day, let alone over a season. The 160F peak temperature is a lot less "pressure" than the 100F night time temperature.
alexqc is nearly correct about the heat storage capacity of the insulation. Different insulation materials have a different "specific heat" (look it up), which affects it's "thermal diffusivity" (look that up too), but at steady state heat flux the net thermal mass (storage capacity) of the insulation or other materials doesn't really matter.
The function of the insulation is to impede heat transfer to a lower rate, not store heat for re-release later. But when there are (160F - 100F) 60F diurnal temperature swings in the attic the thermal diffusivity of the insulating material can lower both the peak and average heat flux through the material, and inserts a useful time-delay between peak attic temperature and peak ceiling temperature. Cellulose has quite a much higher specific heat than fiberglass or rock wool, and at high-R can measurably outperform those materials due to the favorable thermal diffusivity effects.
These thermal mass/diffusivity effects with a time varying top side temperature are explained ( at non-technical level of understanding) in this bit of marketing fluff from a wood fiberboard insulation manufacturer here:
https://gutex.de/en/product-range/product-properties/insulation-in-summer/
>"An analogy. Say you are standing under a faucet that is pouring water on you. You then take a bucket with a small hole in it and put it between you and the faucet -- this reduces the water flow. In the first case, you'd get soaked very quickly and the in the latter case, it will take longer to get soaked... but you will be equivalently soaked in either case, eventually."
Not if the dripping is slowed to the point where you can evaporate the water at a rate that prevents you from getting soaked.
So imagine different sized holes in the bucket and what it'd take to maintain dryness. With a big hole you'd need a very powerful dryer because you'd have a large volume of water pouring on you. With a smaller hole you'd need, let's say a hair dryer. And with a tiny, tiny hole that barely drips at all, you might get away with an incandescent light bulb to keep dry. Now think of the hole size as the insulation level (small hole = high insulation) and the dryer as an air conditioner (light bulb = smaller air conditioner that uses less power).
I don’t follow your analogy here. Remember that if you’re incorporating phase change (evaporation), then you’re doing more than just changing the rate of heat flow.
In a typical system, insulation acts to slow the rate of heat flow from the hot side to the cold side of an insulated enclosure. For the sake of simplicity, let’s only consider the case of heating the interior to maintain a constant temperature when the exterior is cold — like a house in winter. The air conditioning situation is exactly the same, just in reverse.
For every hour, you replace the heat lost through the enclosure to the exterior. You put in enough energy to maintain your constant target temperature inside the enclosure. The insulation acts to slow the loss of heat, which means less energy needs to be put into the enclosure each hour to maintain the target temperature because less energy was lost. In this way, the insulation acts to reduce the amount of energy consumed to maintain that target temperature.
Infinite time scales don’t matter here, only finite time scales (an hour, a day, etc) matter. I think that’s where your confusion is coming from. It’s simple to demonstrate this experimentally in a lab setup, and everyone gets to see it in real life in their homes too. Open a bunch of windows in the winter and your furnace runs more to replace the lost heat, resulting in more energy use and a higher bill at the end of the month. Closing the windows is like adding more insulation, slowing the loss of heat, reducing the amount your furnace runs, and reducing your energy bill at the end of the month.
Bill
Okay, based on the many excellent replies, I can say that one fundamental error in my thinking was that the insulation would be essentially temporarily storing the thermal energy and simply doling it out at a slower rate. Most insulation has very little thermal mass or heat capacity, though, and so it's wrong to think of the insulation "storing" heat at all. A better way of thinking about it, maybe, is to imagine insulation as a conduit of heat that simply throttles any heat on its way through -- but not very much heat ever enters into the insulation in the first place because even the entrance to the insulation is throttled.
But let's look again at my super-hot attic. From what I've read, up to 80% of the heat in the attic is a result of radiant heat (with convection making up most of the rest). This energy radiates into the insulation and then... what? If the insulation can't store much of it due to its low heat capacity, then what happens to that heat? Energy can't be destroyed, so it has to be there somewhere, but where?
I think that's why I kept thinking that insulation had to absorb the heat -- where else would it go?
The energy doesn't radiate directly into your attic insulation but into your roofing and sheathing and then is re-radiated into your attic air. From there a bit will be transferred inside your house by conduction and by convection. The rate at which it does depends on the amount of insulation on your attic floor and how airtight your house ceiling is. The rest of the heat will dissipate outside mostly by convection if your attic is vented. You can think of the outside as an infinite heat sink.
Hot air rises and therefore cold air will replaces the hot air in your attic through your soffit and in the process will evacuate the heat. This type of flow is called natural convection.
I like the way you're thinking this through.
>"This energy radiates into the insulation and then... what? If the insulation can't store much of it due to its low heat capacity, then what happens to that heat? Energy can't be destroyed, so it has to be there somewhere, but where?"
The insulation will get hotter, and it will then dissipate that heat via all available transfer mechanisms (which includes 'through' the insulation to the other side).
So if the sun is directly radiating onto a piece of foamboard, for example, the temperature of the foam on the sun-side will increase, which will create a larger temperature gradient, thereby increasing total energy through-put.
But, in comparison to a highly conductive material, the RATE of that throughput will still be reduced.
So, what happens to the excess energy that isn't 'put through' because of this reduced rate? It is rejected and/or dissipated through all available heat transfer mechanisms: by re-radiating the heat (the insulation, now being hotter, will radiate at a higher energy state), by convective cooling (air will now be relatively cooler than the insulation with higher temperature) and by conduction. Depending on the surface of the insulation, radiation can also be initially rejected (reflected).
>"So, what happens to the excess energy that isn't 'put through' because of this reduced rate? It is rejected and/or dissipated through all available heat transfer mechanisms: by re-radiating the heat (the insulation, now being hotter, will radiate at a higher energy state), by convective cooling (air will now be relatively cooler than the insulation with higher temperature) and by conduction. Depending on the surface of the insulation, radiation can also be initially rejected (reflected)."
The "excess energy" that is neither absorbed by or passing the insulation remains in the attic. Some of it is convected to the outdoors (in a vented attic), or conducted through the roof deck convected away by the (much cooler) outdoor air, and some of it re-radiated back at the sky from the roofing. The radiation temp of a dry clear sky is much MUCH colder than the outdoor roof temperature, and when the sun isn't beating on it the roof temp will drop below the outdoor air temperature (often down to the dew point of the outdoor air on very clear nights.)
Dana, my example was a piece of foam board sitting in the sun, being exposed to an influx of radiation. I can't tell if what you've wrote is in disagreement to what you quoted, or just clarifying? It's not a steady-state, perhaps is your point?
My main point: Temperatures will seek equilibrium. Insulation slows the rate of energy transfer, via that particular path. But equilibrium is always being sought, via all available heat transfer mechanisms (this still includes the insulated path, at least until we invent 'perfect' insulation) ;)
In the case where energy is being added to a system, energy will 'build-up' (or be 'excess') in so far as certain transfer paths have been throttled, slowing the march towards equilibrium (but the march continues).
I would present the water vessel analogy as follows: don't use glass, but rather a substance with some permeability and absorptivity. The permeability of the vessel is akin to thermal transfer. The absorptivity is akin to heat capacity. They both have time effects, but not absolute effects. If outflow is throttled to a rate below the faucet inflow rate, water will eventually spill over.
A thought experiment: If we had a constant heat source surrounded by an air-tight rigid-foam box, would not the insulation eventually pass energy at the same rate as a same-sized air-tight box that is more conductive? There would obviously be a lag as the insulated box gains heat, but eventually, it will become hot enough that the delta-T would allow pass-through of energy at the same rate. Same as how the water vessel will eventually overflow and spill water at the same rate as the faucet itself.
In this sense, insulation only works because the earth spins. ;)
>"Dana, my example was a piece of foam board sitting in the sun, being exposed to an influx of radiation. I can't tell if what you've wrote is in disagreement to what you quoted, or just clarifying? It's not a steady-state, perhaps is your point?"
The foam board box isn't in a vacuum- it is also surrounded by air, which is convecting heat away, and it's still radiating back at the sky. The solar reflective index of the foam will vary by type and any facers it might have- it's not a black-body absorber.
>"In the case where energy is being added to a system, energy will 'build-up' (or be 'excess') in so far as certain transfer paths have been throttled, slowing the march towards equilibrium (but the march continues)."
The equilibrium state is when the reflected, radiating, convected and (smaller fraction) conducted heat equals the rate of incident solar radiation. Most of the time the sky's radiant temperature is near (sometimes below) the freezing point of water, so even a fairly solar-spectrum absorbent (low SRI) roofing material with still air (minimum convection) doesn't keep heating up. It will only reach the temperature at which the radiation back at the cold sky plus the amount of heat being passed through the insulation equals the absorption rate of the incident solar radiation. When you insulate under the roof, the roofing has to hit a higher temperature to achieve that equilibrium, but it WILL be in equilibrium.
When the insulation is at the attic floor, the attic temp rises, and the roof temp rises, but the flux of heat making it through to the ceiling drops. The "extra" heat is being redirected outdoors by the somewhat higher temp of the attic & roofing, increasing the convective and radiant cooling of the roof deck. Going a step further by adding sheet of highly IR-reflective material (say, aluminized fabric radiant barrier) under the rafters, but above the attic floor insulation raises the temperature of the roof deck for higher sky radiation, and the higher temp in the rafter bay channels would increase the drive of convective cooling of the roof deck on the underside, if properly vented from soffit to ridge.
That sort of RB approach lowers the attic's airr temperature while increasing the roof deck temperature, but the overall heat rejection isn't necessarily "worth it" compared to adding deeper insulation on the attic floor (the exception being if there are uninsulated ducts & air handlers in the attic, above the attic floor insulation.)
>"A thought experiment: If we had a constant heat source surrounded by an air-tight rigid-foam box, would not the insulation eventually pass energy at the same rate as a same-sized air-tight box that is more conductive?"
Sure, but so what?
The amount of time it takes for that equilibrium point to be reached is a function of the R-value of the box, and the total thermal mass of the box and it's contents.
You live in an insulated box that is coupled to the earth on the bottom (a very large thermal mass), at a much lower temperature, and are pumping heat out of the box with a heat pump (air conditioner).
The BTU/hr rate the heat pump needs to run at needed to maintain a lower temperature is dramatically reduced by the R-value of that insulated box.
Thanks Dana. I agree with pretty much everything you're saying.
>"It will only reach the temperature at which the radiation back at the cold sky plus the amount of heat being passed through the insulation equals the absorption rate of the incident solar radiation."
Yes I agree. Always tending towards equilibrium. I didn't intend to imply it would continually increase temperature regardless of environmental energy exchange. Quite the opposite. My words may be stumbling.
>"Sure, but so what?"
A fair question. It's not representative of how buildings operate, nor is it intended to be. It was an attempt to address a fundamental concept that Kurt seems to be bringing up. Namely, if insulation slows heat transfer, doesn't that heat build-up somewhere? Where does it go?
My thought experiment was to show that heat will indeed pass through the insulation, and can even do so at the same rate as a lesser insulated system given a higher temperature gradient. It is an example of when a 'build-up' of heat caused by insulation can have a feedback that eventually causes energy pass-through rates (better term?) equivalent to the lesser insulated system.
What I should have expanded upon was that this example is not representative of building climate control systems. There, we seek a constant temperature, rather than provide a constant energy source. I see why it would add confusion, but I felt Kurt was thinking along these lines.
I may have understated that when energy has been slowed from passing through insulation, it will ultimately be dissipated throughout the open system of the environment, according to the laws of thermodynamics. The only thing the insulation really changes is that that particular (insulated) path poses an added hindrance to the energy flow via that path. In addition to convective and—usually to a lesser degree— conductive mechanisms, this includes the radiation to 'cold sky' as you put it (really just radiation, anywhere and to anything). You've added much more specificity on this matter in regards to attics. I'm speaking very generally.
The 'earth spinning' comment was abstract, but only to hint at concepts regarding the larger system exchange of energy and entropy. Without system components that work to (locally) decrease entropy, insulation would eventually be useless. The earth spinning perhaps isn't the thrust, so much as our overall relationship with a distant ball of fusion and the coldness of space surrounding.
I think I'm seeing the discord. Taking a look at Kurt's question:
"This energy radiates into the insulation and then... what? If the insulation can't store much of it due to its low heat capacity, then what happens to that heat? Energy can't be destroyed, so it has to be there somewhere, but where?"
I can see why my response was confusing and/or missed the mark. It is true, as Alex and Dana pointed out, that not ALL this energy is radiated into the insulation. It radiates in every direction, is carried away via convection through venting, and conduction.
It seems as though Kurt is picturing insulation as a sort of dam on a river, where water is backing up behind it. In this case, water will indeed eventually spill over.
My 'thought experiment' is essentially an example of this. Given a constant, steady energy source (like a perfect river), throttling the outflow rates will only delay, and eventual overflow will occur.
Energy does NOT continually build behind our walls, the way a steady flowing river would behind a dam, however. Perhaps shifting the analogy to tides rather than a river would clear this up?
Insulation slows the passage of water when it's high, above the level of your dwelling, until... voila, the water recedes (to varying degrees daily, seasonally, and locationally)—all the while we are running our pumps to pump out what water did make it in. The more waterproof the 'dam' the less the pump has to run.
In energy terms, 'water receding' is simply dissipation into the larger environment (via convection, conduction, and radiation). In an absolute and ultimate sense, this means eventual dissipation into space.
Once the temperature inside the attic is hotter than outside heat flows from the inside to the outside. This happens as while the sun pours more heat in. An equilibrium is reached where the same amount of heat flows out as in, and the temperature inside ceases to rise. When the sun's energy weakens, more heat flows out than in and the temperature inside falls. The delta t is now lower so the rate of heat flow falls and a new equilibrium is established until the sun's energy changes again.
There are cases (eg soil) where a material is a significant insulator and has significant thermal capacity. This causes many to analyze it incorrectly.
It might also be worth mentioning that not all common insulating materials have the same thermal capacities relative to R-value. Cellulose, for example, has greater thermal capacity than foam board, which may subtly affect the characteristics of an envelop. They also have different moisture buffering capacities.
It may be useful to considider steady-state conductive heat transfer as an analogy with electrical current flow. A current will "flow" through a resistor at a given voltage difference with a magnitude proportional to the magnitude of the resistor. Heat flux density will occur at a given temperature difference in proportion to the connductivity of a given material. Heat flux occurs via transfer of electron vibrations. Air, as most gases, is a poor conductor (good insulator; better than any common solid insulator). Insulation works by creating small pockets of still air. Why use insulation at all if air is so good? If air is not confined to small pockets, convection becomes the primary heat transfer mechanism and it is very effective. Hence air sealing is key.
>" Namely, if insulation slows heat transfer, doesn't that heat build-up somewhere? Where does it go?"
The heat "goes" back from whence it came (sort of).
By blocking/slowing the transfer of heat the warm side of the assembly runs a bit hotter, since it isn't passing through.
At the higher temp is is radiating more heat at the cold sky...
...and convecting more heat into the proximate local atmosphere.
There is nothing mystical or mysterious going on. Under incident solar radiation the roofing temperature is normally several 10s of degrees F hotter than the surrounding air, and the attic temp under the hot roof deck is a few 10s of degrees cooler than the exterior roofing temp, also above the outdoor air temp.
When there is ZERO insulation on the attic floor in an air conditioned house the attic temperature runs a degree or three cooler than when it's insulated, since the heat is being transferred to the conditioned space at a high enough rate to measurably affect the attic temp. Insulate the attic floor to R30 and that cooling effect is much reduced- the attic temp rises a few degrees, as does the roof deck temperature, sending more heat back to the exterior environment via convection & radiation than the uninsulated attic situation.
I have a similar question:
I have a wet underfloor heating system embedded in a screed. On top of the screed I have a plywood base and oak floorboards. The oak floorboards were kiln dried to 6% moisture to avoid movement.
My heating is provided via a ground source heat pump. My heating bills have been much higher than those predicted when the gshp was installed. The installer says that this is probably due to the thickness of the wooden floor covering, rather than any problem with the gshp system.
My take on it is that the thickness of the wooden flooring shouldn't have any (or more than minimal) effect on energy usage. It just means that the floor takes longer to heat up when first turned on. Since I have the heating on all the time (in winter) I don't see how the thickness of the wooden floor should make any difference. The extra thickness will eventually heat up to the requisite temperature.
Foldyard,
Edit: The R-value of hardwood is about R-0.7 per inch. I can't see how that would make an appreciable difference to the efficiency of the system.
The effect of the insulation value of the floor is not to slow the responsiveness -- that is a function of the heat capacity of the floor, and most of that is going to be in the screed, which was planned for.
The effect is that in order to get the same surface temperature -- which is what determines the heat output -- your water has to be hotter. Yes, your system is going to be slightly less efficient with hotter water, but it's going to be really marginal. So I think he's full of it.
If the system is using more energy than projected, there really are only two possibilities: either the system is consuming more electricity than projected to produce the heat needed to keep the house warm; or the system is producing more heat than projected, but either only part of it is going to heating the house, or heating the house takes more energy than projected.
With a system like this you can measure instantaneously how much heat it is putting out. If you take the flow rate in gallons per minute, multiply it by by the temperature difference between the outgoing and incoming water, and multiply that by 500, you get the heat output in BTU/hr.
You can measure the instantaneous electricity consumption with a clamp-on ammeter, which will tell you if the system is getting the efficiency that was projected. This would usually be expressed as COP, coefficient of performance.
You can compare the heat production to the projected heat load on cold days to see whether the house is taking more heat than was projected.
Rather than continuing to tack onto a thread that's over three years old, could you start a new thread with your question? Thanks.
Thanks
I found this reference, which probably answers my question about the impact of thickness on cost:
"The flow temperature depends on the required output and the chosen flooring. Normally, the flow temperature is 30 to 45 °C, and it should not exceed 50 °C. The flow temperature has a limited impact on the heat energy consumption. If the temperature is raised from 30 to 45 °C, heat energy consumption only increases by 6%, as energy consumption is determined by the difference between the flow and return temperature. It is only marginally more expensive to heat a thick plank floor than a thin engineered wood floor, but it takes a higher flow temperature to ensure the required surface temperature."
In introductory physics one of the concepts that is taught is the difference between heat and temperature. Much of introductory physics is about teaching the student that his intuitive concepts about the physical world are often wrong, and intuitive notions about heat and temperature are often among them.
Readers of this website know that I try to discourage the use of the term "thermal mass"; it's not a term that is used in science and often is used by people who are confused about the science. One of the things I object to is the adjective "thermal." "Thermal" is problematic because it can refer to either heat or temperature. Mostly it's used to describe temperature effects -- thermal expansion, thermal stress -- but it is used to describe heat sometimes. Reading through this thread "thermal" is used a lot -- 34 times -- and it's mostly used incorrectly.
There's no such thing as "thermal energy." That's heat. Call it heat. That's the scientific term. There's no such thing as "thermal capacity." That's heat capacity or specific heat depending on what you're measuring. "Thermal transfer"? Nope, heat transfer.
And of course there's no such thing as "thermal mass."
I am interested in what say about heat and temperature and intuitive concepts being wrong. Could you possibly expand on this? Thanks
Sure. The most common intuitive mistake is to think that hot things have a lot of heat, and things that aren't hot don't have a lot of heat. If you've ever spend time on forums the classic post is along the lines of, "I don't understand it, my solar collector is at 160F but it struggles to heat the house above 50F." That's confusion between temperature and heat.
Insulation slows down heat transfer, like a speed bump for heat. It might not stop it entirely, but it gives you time before it gets through. In cooling climates, insulation is crucial because it helps maintain a comfortable indoor temperature. It doesn't have to stop all heat, just slow it down enough for your HVAC system to keep up.
Experts: Clarify and correct as needed...
I used to live in a stone house with 20" walls, which provided a lot of thermal mass. In Spring, on the first hot day the house stayed cool. Likewise in the fall, the house remained warm for day or two even if the outside temps had suddenly dropped into the 40s. But during a long hot August, that thermal mass was no help.
It took a couple of days for that old stone house to reach its a point of energy equilibrium with the outside. But stone is not a good insulator.
Thanks, Dana for suggesting we lookup thermal diffusivity. So even though stone may be fairly conductive and a lousy insulator, massive stone houses can be comfortable, at least for a short time, because as the formula for Thermal Diffusivity (shown on Wikipedia) elucidates the density and specific heat capacity of the stone material lowers the system's ability to transfer heat.
What about those traditional South Western adobe structures? I'm not very familiar with the materials used to make a traditional adobe home, but I suppose traditional builders incorporated natural insulating materials, such as straw which entrapped air pockets in with the dried mud. Exactly how did adobe help keep ancient folks from roasting in a long Southwest summers? Maybe adobe is awesome: some thermal mass to smooth out fluctuations in heat; plus straw and entrapped air pockets to slow the conductivity of the mud - all in a package of naturally found materials.
Sometimes I confuse CONDUCTION vs CONVECTION, so it was helpful to see the following emphasized: conduction involves the transfer of energy through a SOLID, while convection is the transfer of energy through a FLUID: gas & liquid.
From the webpage: https://www.sciencelearn.org.nz/resources/1006-insulation
"Heat is lost in one of three ways – in each situation, heat moves from a hotter object to a colder one:
" Conduction – the transfer of energy in a solid object from one end of the substance to the other by the rapid vibration of atoms.
" Convection – the transfer of heat energy through a liquid or gas by the movement of the fluid (atoms or molecules). This movement, caused by the expansion of the fluid, produces a current as its temperature rises, called convection current.
" Radiation – the emission of any rays, wave motion or particles from a source, most commonly applied to emission of electromagnetic radiation.
Pockets of Air
One hears a lot about how insulation has "pockets of air", which help prevent (or more accurately to slow?..) convection, thereby slowing heat transfer. Besides inhibiting convection, Insulation's solid bits are probably poor conductors. I am always wondering how exactly do those "pockets of air" help stop overactive air molecules sharing energy with their cooler neighbors?
I read a question on Reddit: Why if air is considered a good insulator, do we fill insulation with stuff other than ... just air? A writer replied: air is a poor conductor, but an excellent convector.
Adobe houses work well much of the time because of the typical, large temperature swings between day and night. It might be 90°F during the day but 60°F at night, so the walls tend to average around 75°F. If it remains hot for a night or two, or daytime highs are below room temperature for a day or two, the inside won't be comfortable. That's why traditional adobe homes also always have at least one fireplace, often with adjacent benches so occupants can sit close to the warmth because your skin will radiate heat to the cool walls.
Insulation's solid bits vary in thermal conductivity. Mineral wool is composed of rocks, usually basalt, which is a good conductor of heat. Wood fiber is somewhat insulative, at roughly R-1.2/in.. Foam is probably somewhere in the middle. The key is that at a specific size, air becomes a poor convector. In multi-glazed windows, for example, that's around 3/4" if I recall correctly. That may be with argon, not regular air, because argon is a better insulator than air. Krylon is better still, but not by much and it's very expensive. With insulation, it appears that the air spaces can be quite small and still have low convection, so while the solid bits are conductive, the air pockets balance that out.
With foam insulation, it's not air in the pockets but blowing agent, except for EPS and GPS. Like argon in a window, the blowing agent--currently either a hydrofluorocarbon or a hydrofluoruoolefin--is a better insulator (aka worse conductor) than air. But over time, those blowing agents are replaced by air, so XPS' R-5 will eventually be around R-4.3 and polyiso will eventually be around R-5.6. "Eventually" varies a great deal so it depends on the product and installed conditions how long the change will take. It could be years, or it could be decades.
Bingo.
I was in New Mexico in late February/early March this year. I visited a well-preserved Pueblo. The houses were brutally uncomfortable. It was around 30F and windy. Since the houses were meant to be heated with fireplaces they weren't very airtight and the wind just whipped through them. The adobe provides no insulation to speak of.
In addition to providing some heat capacity, the adobe structures also provide shade, which I can see being useful in the heat of the summer.
I am sure they were uncomfortable, but they just wanted to stay alive. Guess we are all spoiled
Krylon? I'm spray paintin' mah windahs......funny couldn't pull 'krypton' out of my head to make fun of you, had to look it up
Haha, I had a vague feeling that I wasn't using the right term but I needed to get back to work so I didn't spend time on it. You're right, of course; I meant Krypton.
> Adobe houses work well much of the time because of the typical, large temperature swings between day and night. It might be 90°F during the day but 60°F at night, so the walls tend to average around 75°F.
And that's 100% why you don't see adobe houses (or other thick-walled concepts) in very hot cities like Phoenix. The "heat bubble" created by the metro area means that the nighttime temperatures never dips below 80°F in the summer and occasionally won't even go below 100°F! That means that those walls will never get a chance to cool down and you'd have the equivalent of one of those in-oven baking stones baking everybody in the house all day and all night long by mid-May.
Nor do you see them in very cold places.
kurt,
Climate-specific vernacular architecture build with what materials were available.
I agree, Malcolm. There are plenty of stone houses in Europe and in the US, where they behave similarly to adobe, for better or worse. And they often have (or had) a fireplace in every room.
Sure, and they weren't always comfortable. In Siberia they built houses out of mammoth bones and reindeer hides and heated them by burning peat. Not how I'd like to spend a Siberian winter.