Warming a cold-soaked house
If a house with a total weight of 150,000 lbs and 2,000 lbs of air needed to be warmed from 60° to 70°F, would it really take 1,520,000 Btus?
If the minisplit output was 32,000 Btu/hr, that would be about 48 hours. Yes?
GBA Detail Library
A collection of one thousand construction details organized by climate and house part
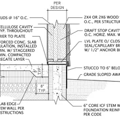
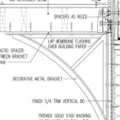







Replies
Mark,
I can't comment intelligently on your figures, but every morning I turn on three baseboard heaters and heat my house from 60F to 70F in about 45 minutes.
You would calculate this by using the specific heat for all the different components of the house, which would likely end up as a sort of weighted average for the overall house. The part that is air is easy. From there, you calculate using the unit of energy which is a “joule” (one watt/second), and you can work out approximately how much energy input you need to raise the temperature 10 degrees.
In reality, it’s much more complicated. You’re not really heating the entire mass of the house. You’re heating a gradient through the walls, for example, since you stop heating when the inside of the house heats up to your target temp. The outside of the wall will be closer to outdoor ambient temp. You basically heat all of the air mass, plus part of the mass of part of the house, and all of the mass of other parts (like interior walls).
I suspect your calculation is simplified (like heating all of the house), maybe assumes a starting temperature of 0*C, and possibly has a unit conversion error (which is very easy to do in those types of calculations), which can easily result in errors of orders of magnitude (10x, 100x, etc). You’ll also find that the real house will “leak” heat (we use insulation to slow this down, but it still happens), which has the effect of increasing the amount of energy you need to put in to raise the temperature.
I would expect it to take much less time to heat up that house 10 degrees than your calculation would seem to indicate.
Bill
This is definitely a theoretical house, not actual and it's not a normal morning routine.
The big assumptions were that the 60° was a steady state and the 70° was a new steady state. Applying heat for 45 minutes will warm the air until that heat transfers to the structure.
Also, I took 1 BTU to warm 1 lb of water 1°F as the same amount as for 1 lb of house. That created a large error. I controlled for (ignored) atmospheric variables (heat loss/gain & infiltration).
Now that you mention it, I see the specific heat of building components to be 1/2 to 1/5 that of water.
Thanks.
Water is rather spectacularly good at holding heat, so if you assumed the specific heat of water for all components that would result in a much higher than actual heating load. Glad I was able to help you a bit with your calculation.
There are some real-world applications for this. In large datacenters, the chillers that make cold water for the cooling plant can’t immediately restart after a power interruption — there is a 10-15 minute delay while the unit recovers. It’s inefficient to put battery backup on the chillers (battery backup systems have around 6% losses 24x7), but the computer servers are backed up so heat generation continues during the chiller restart delay. I have to maintain the datacenter temperature within limits, so I put a large insulated water tank in the chilled water loop. The size of the water tank is determined the same way as your heat load calcs, but the goal is to use the tank as a sort of thermal battery to keep the temp rise of the chilled water loop below a certain limit. We size the tank to absorb the heat output of the facility over a 15 minute time period without the temp rising outside of acceptable limits. This is much more efficient than any other options.
Bill
I did the opposite and found my house uses about 5kW of heat energy to cool by 1ºC
In summer the house gets heat soaked all day with no AC and will only cool about 1ºC all night when the temps are about 5ºC lower at 10ºC its a bit over double.
So what i did was use a window fan and calculate the volume and temperature difference and the rate of cooling. And i did it slowly because the thermal mass does not absorb coolness quickly.
My figure is not exact but its repeatable and its close enough to use as a personal shorthand and i can replicate it by using AC at night given its tonnage rating.
Do you mean you use 5kw/hr?
5kw is a rate, not a unit of energy. 5kw/hr is a unit of energy. 5kw/hr can convert to about 17,000 BTU, since a BTU is also a unit of energy.
17,000 BTU/hr is a rate, so it would convert to 5kw. Either unit in this case is a rate, so in either case to determine an absolute amount of total energy consumed or moved, we need to know how much time the system was operating, in minutes, hours, etc.
This is an important distinction and I see it misused frequently which can lead to large errors, or, worse, misunderstanding and misapplication.
Bill
Sure 5kWh/ºC
5kW/hr isn't a unit of energy, it's a rate per unit of time, kind of like acceleration. The unit you're looking for is kWh.
The thermal mass of the house isn't very closely related to it's mass. The differing materials all have different specific heats, as Zephyr7 correctly points out. A pound of water takes about nine times as much heat to raise the temperature as a pound of iron or steel, 4-5x as much as a pound of concrete, and about 56 x as much as a pound of air.
See:
https://www.engineeringtoolbox.com/specific-heat-solids-d_154.html
And that's before you get to the complexity of how much of that thermal mass is inside the thermal envelope of the house, temperature gradients, etc.
"A pound of water takes .... and about 56 x as much as a pound of air." Minor "oops." On a mass basis, that would be about 4x. The heat capacity of air at room temperature is around 0.25 BTU/lf-F, vs water's 1.0. The number 56 would be for a lb of water vs a cu.ft. of air (density around 0.075 lb/cuft).
I found the thermal mass in our house held about 20,000 Btu's per degree F for whatever this is worth. I have a very good idea of the homes heat loss in BTU's (360) per degree F. I timed furnace run times for 8 hours once the house was up to temperature on cloudy, windless steady (January) state temperature day. The furnace efficiency was known at 95%. The hourly heat loss figure is also backed up by a detailed heat loss calculation, a pressure test and 6 years worth of energy monitoring.
I arrived at the 20,000 Btu figure by watching the indoor temperature fall after 10:00 PM after the thermostat setback. I would then factor in the Delta T for the period and the 360 BTU per degree F heat loss to arrive at the thermal storage capacity for the house.
The house is located in Minneapolis, 3,328 SF rambler with full basement. The construction is standard wood studs and drywall, interior volume is about 29,000 cubic feet. The thermal capacity of air would have to be factored into the calculation, the ACH50 for the house is 3.
Anecdotally, the one time I made a mistake of trying out a setback on an older house (2 story uninsulated double brick) with radiators, it took about 4h to get the house from 15C to 20C with a 75k boiler. If the boiler was firing for the whole time that works out to 300kbtuh.
Edit. Forgot to include the heat loss for the house. My guess is around 30k, which brings the would bring the number down to 140kbtuh.
That would be 300KBTU, or 140KBTU (fixed quantities of energy, not time dependent) not kbtuh (a rate of energy per hour).
Anecdotally, it took 10 days at about 3-4kW to raise my house from 13degC to 20degC. The outside temperature was between -10C and -25C the whole time, so I'm guessing a good 2kW of that was lost to the outside.