R values less than 1 don’t make sense
Let’s say there’s a 100sf wall through which heat can pass unimpeded, and there’s a 30°F difference on one side of the wall vs. the other. The amount of heat that would transfer is:
100sf x ∆30°F = 3000 BTU
Now we add R13 insulation, and get:
100sf x ∆30°F ÷ R13 = 231 BTU/hr
However, instead of adding R13 insulation, say we added 1/2″ gypsum. Various sources put the R-value of drywall at 0.45 per 1/2″. But when you divide by a number less than 1, you get a *higher* number. So:
100sf x ∆30°F ÷ R0.45 = 6667 BTU/hr
That’s more than the amount of heat we started with! Clearly I’m missing something. What am I missing?
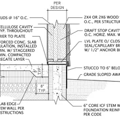
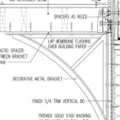
GBA Detail Library
A collection of one thousand construction details organized by climate and house part







Replies
You have the right formula:
Heat flow=area*temperature delta/R-value
Where you're on the wrong track is what the unimpeded wall looks like. That's not R1, it's R0, which would require infinite heat flow to keep the other side at 70F. The model just breaks down when the R-value gets really low.
With R0.5, you'd need 6,000 BTU/hr.
Okay, thank you, I get it now. I erred by thinking that before you divided by R-value, the figure you got was the amount of heat on the other side of the wall. It's absolutely not. It just sets you up to divide by R-value so you can figure the rate of heat transfer.
So, for various R-values:
100sf x ∆30°F ÷ R13 = 231 BTU/hr
100sf x ∆30°F ÷ R5 = 600 BTU/hr
100sf x ∆30°F ÷ R1 = 3000 BTU/hr
100sf x ∆30°F ÷ R0.5 = 6000 BTU/hr
100sf x ∆30°F ÷ R0.1 = 30,000 BTU/hr
100sf x ∆30°F ÷ R0 = divide by zero error
However, all this presumes that there's more heat to transfer than the one-hour transfer rate. For example, if we have a flow rate of 3000 BTU/hr, but after 1800 BTU of heat flows, the source side has now cooled to the same value as the target side (because it lost its heat), then 3000 BTU didn't transfer, only 1800 did. And of course, as the heat was flowing, the difference in temperature between both sides changed, which changes the formula.
A perfect conductor doesn't exist. Your "unimpeded" wall will have some resistance to heat flow. For a wall about the worst you can get would be metal siding. It will have a really low R# greater than zero. Lets say R0.05 for sake of example.
100sf x ∆30°F ÷ R0.05 = 60,000 BTU/hr!!!!
The model assumes a constant temperature on one side, and the other side is effectively and “infinite sink”, that can take al that you can throw at it. This means the hot side NEVER cools off, and the cold side NEVER warms up. The purpose of the model is only to show the heat flow through the insulation, it doesn’t have anything to do with the “stuff” on either side of the insulation. This is a theoretical model after all, and there is the saying “theory is like most on glasses: obscures facts”.
Yes, in reality the warm side would cool off if you were insulating a box with a fixed amount of contained thermal energy. In a practical home though, there is a thermostat trying to maintain a fixed set point temperature, so it will increase the energy input as the insulation’s R value drops off (just as it does when the R value is fixed but it gets colder outside). In this system, you CAN assume a relatively constant indoor temperate, so the model tells you your BTU losses through the wall, which is also the number of BTUs your furnace needs to put into the home for the thermostat to maintain the setpoint temperature.
Bill
Yes absolute R values less than one are irrational. You never have to worry about it because the r value of air layers on either side of the surface are one or greater.
R values less than 1 are definitely a thing and do work. With any type of assembly you'll have some interface layer between the material and exterior conditions, so the effective R value is always higher for example, the OP's original post.
Once you take into account air films, drywall/sheathing and airgap between studs you end up with an R3 assembly even if you have zero insulation.
So using the same numbers:
100sf x ∆30°F ÷ R3 =1000BTU
Insulate with R15 batts, you end up with an R12 assembly once you take into account the thermal bridging from the studs so:
100sf x ∆30°F ÷ R12 =250BTU
So that is still significantly less but you can see when you are dealing with milder climate and low delta T, going much above that R12 is not really worth it unless it can be done for cheap.
Perhaps I was unclear
Certainly r values below 1 work when added to or subtracted to a number such that the end result is a number higher than one.
Total r values under 1 become irrational.
1000 sq ft Delta T 50 R1 5000 btu
other than a lab condition with hurricane force winds on both sides I cannot think of any situation where in air you would get higher heat transfer than that R 1 number.
For example if someone tries to say that a material has an R value of .09 it does not follow in the real world that suddenly that surface is suddenly going to see 55555 btus of heat transfer
R-values below 1 are no problem. It's R-values equal to zero or less that are a problem.
R1 is not zero insulation. It's about a quarter inch of fiberglass or open cell foam.
no, that would be about r 2
because of the air layers
As a practical matter, if a surface is closed, the air film has an r-value. For the exterior it's assumed to be 0.17 and on the interior 0.68. So a wall that is air-tight but has no insulation -- think a sheet of sheet metal -- would be r-0.85.
Those air film R values are for still air though -- moving air cancels some to most of that out.
I'm not sure why the confusion about low R values though. Anything over R0 is still insulation, and will reduce heat flow. You have to remember that if you're comparing to zero insulation, which is theoretically infinite heat flow, you'll see big numbers for BTUs moving at low R values, since you're approaching a limit. Fun math stuff. The models assume infinite source and sink ability for producing and removing BTUs, so you can get whacky-looking numbers when running theoretical exercises like this. That doesn't mean the model is wrong in this case, you just have to understand what's going on.
Bill
" other than a lab condition with hurricane force winds on both sides I cannot think of any situation where in air you would get higher heat transfer than that R 1 number."
Why?
There's nothing special about r-1. The r-value scale was created to make the math easy, the units are arbitrary. In countries that use the metric system they have a different r-value scale, r1 there is not the same as r1 here.
What is the same everywhere is r zero. Zero insulation is zero insulation. And yes, the formula tilts at r zero, there's no such thing as infinite heat
There is nothing special about R1, it is is just a practical impossibility to reach it in a building
air layers
small amounts of air movement do not eliminate the air layers
do the math and explain to me where on this planet you have ever seen R0
What you do see is R1
Like a single pane of glass
So what happens in metric countries where they express R-value in kelvin square-metre per watt instead of degree Fahrenheit square-foot hour per British thermal unit? And their R1 is our R5.68? Is it still a practical impossibility to reach it?
yes
[which is what I have been saying all along]
If I had to estimate the R-value for an insulated pole barn -- sides and top are just a few hundredths of an inch of galvanized steel -- I'd say that the steel is effectively zero, the inner air layer is 0.68, the outer air layer is 0.17 and the assembly R-value is R 0.85.
If you've ever spent time in one on a sunny summer day you know they have some capacity to hold heat. If you've spent time in one in the winter you know it's not much.
For heat transfer calculations, "R" is defined as the reciprocal of "U," the heat transfer coefficient, which does have units. For unidirectional heat transfer, since the reciprocal of overall U is the sum of the reciprocals of the individual U values of the layers, the math does indeed get simpler by using R values. Those are additive, by definition.
One place I understand very low R values make a difference
It is my understanding one of the differences between a 92 percent hot air furnace and a 96 percent hot air furnace is the thickness of the sheet metal.
Stainless steel is a relatively poor conductor. They make the sheet metal thinner to make up for it in a very high efficiency furnace.
But what do we know about a furnace? There is:
A very high Delta T [Making small changes matter]
There is a large fan blowing directly on the outside[air layer]
There is a fan forced flame running on the inside[air layer]
So in this very particular case the engineers have no doubt calculated that difference at a very low R value.
I think the issue is that gus is simply claiming the R values below 1 don't practically exist in building assemblies. Others are arguing the fundamental math of it. It's two different questions.
I think it would have helped if gus did not phrase it as "R values below 1 are irrational."
In my opinion, It made it sound like he thought the math was invalid (irrational not having any real meaning in its use, but to me implying the calculation itself was invalid).
Perhaps saying "total assembly R values below 1 are unlikely to exist in common building assemblies" would be clearer.
Honestly, I still think that's what he is saying. If you look at replies 14 and 16, he says that R5.68 is also irrational (but only when you convert it to metric). It somehow changes the physics when you change the units. I wonder how the wall knows what units it's in.
What happens in Canada where they interchange metric and US units?
Maybe he can clarify his position.
Martin thinks he's the energy nerd, but y'all have created an epic debate about the technicalities of R-values.