Effects of tapering insulation at eaves
I am trying to quantify the effects of insulation tapering off as you get closer to the eaves. eg. R-49 in the main attic, but the final 2 ft as you approach the top plate taper off to lets say R-19 instead of blocking eaves and giving full R-value to the edge.
I can run the heat loss calculation pretty easily for 2 separate attic areas of different R-value, but can’t find a calculation for slowly tapering it off.
I am trying to help quantify for customers that the extra time is worth the effort. (which I believe it is, just needing to quantify)
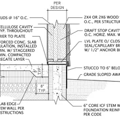
GBA Detail Library
A collection of one thousand construction details organized by climate and house part







Replies
Deleted
I would think that for a linear taper the average insulation would be pretty accurate, IOW assume R34 for the entire area that is tapered
Averaging the R values doesn't work for rectangular sections, and I don't think the triangular section affects that.
For example, compare two rectangular sections:
- One is R-34 all the way across
- One is half R49, half R19
You have to average the U values (not the R values), so Uavg for the half and half scenario is:
(1/49+1/19)/2 = U-0.036, for an Ravg of R-27.
I'm not sure quite what the correct way to approach a triangular area is with regards to average r values though.
My intuition is that the effective R-value of the triangular area tapering from R-49 to R-19 is higher than R-27 but lower than R-34.
Energy vanguard has an article about this issue: https://www.energyvanguard.com/blog/25547/Flat-or-Lumpy-How-Would-You-Like-Your-Insulation
R31.7 (by analyzing it in terms of heat loss over every micro inch).
I'm not sure I buy into the linked article. not enough science in there
Let us look at this situation, a percentage of the roof is under insulated.
were it a 24x40, about 17 percent is under insulated by.....some percentage.....so if you over insulated the majority of the roof by a bit............
Are you talking about the article I linked? What don't you buy?
My reading of the article is that it is simply pointing out the fact that uneven insulation is less effective than the same amount of insulation spread evenly, due to the fact that you have to average the U-values not average the R-values to figure out the performance. It didn't seem particularly controversial to me.
Heat moves to cold, "over insulating" serves no benefit if all the heat is moving to the under insulated portions.
As I have been doing the manual calculations on the Heat Loss by essentially stair stepping the insulation every 6 inches dropping by R-5
In your 24x40 example that 17% that is under insulated would loose roughly 32% of the total heat through the ceiling.
Jeff,
Not wanting to complicate things unnecessarily, but there are several other considerations beyond the straight calculation of loss of insulation that come into play.
Probably more than any other area on the building envelope, the area over the top-plate is prone to poor air-sealing and wind-washing of the insulation, which further degrades the R-value.
The lowered insulation value at the eaves can contribute to both ice-dams above, and moisture at the intersection of the wall and ceiling below.
I completely agree that these additional factors come in to play. For the average homeowner I wanted to isolate the pure R-value numbers, and then separately address the air sealing issues.
Jeff,
That's probably a really good idea. Conflating the two would leave most homeowners quite confused.
When I did it for my own house, I used the formula for finding average u-value. Uavg = (U1 x A1) + (U1 x A1) + etc./Atotal
A = Area
U = U-value
I just sectioned it off into 1 inch increments and calculated the area for a 1" x 12" section. Then you multiply that area by the decreasing U-value for each 1" section, as the roof deck gets lower. This will change depending on your roof pitch and heel height. Then you can multiply the end result by the perimeter of your roof. Input that and the area of your full R-value roof into the Uavg equation if you want to know how it affects your overall ceiling R-value.
There is probably a faster way, but that worked for me.
Interestingly enough, it amounted to about 17.5% more seasonal heat flow through my ceiling. R50 to R42.5 average. Or about $15 in propane heating.
Good enough - every inch yields R31.0 (vs the correct R31.7).
Out of curiosity Jon, what did you use to arrive at that R31.7? Formula or software, or...? If there is a faster/better way, I'd like to know it!
3 lines of software. I suspect there is a formula, but I can't think of it.
edit:
for (int i = 0; i < STEPS; ++i)
U += 1./STEPS * (1./(BASE_R + (i * ADD_R/STEPS)));
printf("result = %f %f\n", U, 1/U);
Not to be a bother but...what software? :D
This is reaching way back to math class long long ago and I don't feel super confident remember how to solve this, but I got the same answer Jon did...
If x is the distance from the eave,
R-value at x = 1.25x+19
U-value at x = 1/(1.25x+19)
If you get the integral of 1/(1.25x+19) from 0 to 24, that's the area under the curve. Then divide that by 24 to get the average u-value.
Using an online integral calculator, the integral is:
Utotal = 0.7579050551553489
Uavg = 0.7579050551553489 / 24 = 0.03157937729
R = 1/0.03157937729 = 31.67
That should get you the precise answer right? Any mathy folks wanna confirm this?
Wow, I'm rusty. Building on Brendan's answer, I can generalize it for any linear change in R value:
Effective R = 1/(ln(49/19)/(49-19))
where:
49 is the max R value
19 is the min R value
Notice that the distance (24") has dropped out.
Nice! Thanks Jon and Brendan!