Effective insulation
What is the effective insulation value of a 2×6 stud, 16″ center wall with spray foam? I can find the R value of the stud – 6.88, and the R value of (open-cell) spray foam at 5.5″ = 19.8. But it seems that it is a calculus problem to figure out the effective R value since the stud has a lower R and so provides an easier path for heat transfer for at least some of the space between the studs.
Thanks!
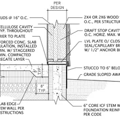
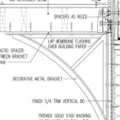
GBA Detail Library
A collection of one thousand construction details organized by climate and house part







Replies
Steve, see this link: https://www.greenbuildingadvisor.com/article/free-online-calculators-for-r-values-and-costs
Steve, also see https://www.greenbuildingadvisor.com/article/installing-closed-cell-spray-foam-between-studs-is-a-waste
1.) The Oak Ridge link is no longer available.
2.) It is not just R1+R2; I can figure that out -- for heat closer to the stud than 5 1/2", it can travel horizontally and then go through the stud more easily than through the insulation which is only 5 1/2" deep. So out of the 16" between studs, the overall R value is less than the R value of the insulation taken by itself. There is a distribution where the maximum R value is that of the foam insulation, but outside of a middle ~ 5" it is less than that. Surely someone has done this calculation.
And, actually I am Mary Hoyer.
Maybe 14.02, 16.0 including everything. You can use this tool. It will also give you durability ratings.
http://effectiver.ca/calculator/wall.php?id=11846
http://cwc.ca/resources/effective-r/
Mary Hoyer,
One of the links that Bill Dietze provided ("Installing Closed-Cell Spray Foam Between Studs is a Waste") has the answer to your question. Here is the relevant section from that article:
To calculate the whole-wall R-value of a wall, you first need to calculate the whole-wall U-factor. (U-factor is calculated from R-value this way: U=1/R.) Let’s call the U-factor of the insulation UI, and let’s call the U-factor of the framing UF. Here’s how we calculate whole-wall U-factor for a wall without any windows or doors:
Whole-wall U-factor = (UI * percentage area devoted to insulation) +
(UF * percentage area devoted to framing)
Thanks, but that doesn't take into effect the thermal bridging that presents a second path for thermal transfer, which is the question that I was asking.
I'll try the calculator, but that looks like it is just additive as well.
Mary,
The formula Martin provided accounts for thermal bridging for the layer you plug in the values. You have to do a U-value calc for every layer, then add them up. The calculator here does that all for you, and allows you multiple different layers, and does the percentage framing calculation for you.
https://ekotrope.com/r-value-calculator/
Mary,
I know I probably shouldn't ask this on a site edited by a self-confessed energy nerd, but why does it matter? The level of accuracy you are asking for isn't useful when designing or building a house.
Mary,
As Steve pointed out, the formula I provided does account for thermal bridging through the framing -- by taking the U-factor of the framing (which is usually a higher U-factor than the insulation) and multiplying that U-factor by the percentage of the area devoted to framing.
That's what accounting for thermal bridging is all about -- accounting for the elements of an assembly that have a higher U-factor than the insulation.
The cwc.ca data seems to indicate that the formula/data is (for some reason) inaccurate by 20%. Other sources indicate that for wood frames, parallel path calculation errors are around 2% (much much higher with metal framing).
An easily to understand case where 1D analysis fails is comparing the net effective R value of a steel bolt through a steel sip vs the same bolt through an OSB sip (the former bolt moves more btus, despite being the same % of area).
Mary, if you look at isotherm diagrams, which show heat flow through assemblies, you can see the effect you're describing: the cavity insulation near the studs is somewhat cooler than at the center of the framing cavity. But the studs benefit from the opposite effect, and are warmer at the edges than at the center. In Passive House training you learn how to account for thermal bridging at inside and outside building corners, but for walls, adding up the R-values at each wall segment is the standard approach. A higher degree of accuracy would require some complex calculations, best left to computers.